df?integrate1d/df?integrateex1d

Computes a spline-based integral.

Syntax

FORTRAN:

status = dfsintegrate1d(task, method, nlim, llim, llimhint, rlim, rlimhint, ldatahint, rdatahint, r, rhint)

status = dfdintegrate1d(task, method, nlim, llim, llimhint, rlim, rlimhint, ldatahint, rdatahint, r, rhint)

status = dfsintegrateex1d(task, method, nlim, llim, llimhint, rlim, rlimhint, ldatahint, rdatahint, r, rhint, le_cb, le_params, re_cb, re_params, i_cb, i_params, search_cb, search_params)

status = dfdintegrateex1d(task, method, nlim, llim, llimhint, rlim, rlimhint, ldatahint, rdatahint, r, rhint, le_cb, le_params, re_cb, re_params, i_cb, i_params, search_cb, search_params)

C:

status = dfsIntegrate1D(task, method, nlim, llim, llimhint, rlim, rlimhint, ldatahint, rdatahint, r, rhint)

status = dfdIntegrate1D(task, method, nlim, llim, llimhint, rlim, rlimhint, ldatahint, rdatahint, r, rhint)

status = dfsIntegrateEx1D(task, method, nlim, llim, llimhint, rlim, rlimhint, ldatahint, rdatahint, r, rhint, le_cb, le_params, re_cb, re_params, i_cb, i_params, search_cb, search_params)

status = dfdIntegrateEx1D(task, method, nlim, llim, llimhint, rlim, rlimhint, ldatahint, rdatahint, r, rhint, le_cb, le_params, re_cb, re_params, i_cb, i_params, search_cb, search_params)

Include Files

Input Parameters

Name

Type

Description

task

Fortran: TYPE(DF_TASK)

C: DFTaskPtr

Descriptor of the task.

method

Fortran: INTEGER

C: MKL_INT

Integration method. The supported value is DF_METHOD_PP.

nlim

Fortran: INTEGER

C: MKL_INT

Number of pairs of integraion limits.

llim

Fortran: REAL(KIND=4) DIMENSION(*) for dfsintegrate1d/dfsintegrateex1d

REAL(KIND=8) DIMENSION(*) for dfdintegrate1d/dfdintegrateex1d

C: float* for dfsIntegrate1D/dfsIntegrateEx1D

double* for dfdIntegrate1D/dfdIntegrateEx1D

Array of size nlim that defines the left-side integration limits.

llimhint

Fortran: INTEGER

C: MKL_INT

A flag describing the structure of the left-side integration limits llim. For the list of possible values of llimhint, see table "Hint Values for Integration Limits". If you set the flag to the DF_NO_HINT value, the library assumes that the left-side integration limits define a non-uniform partition.

rlim

Fortran: REAL(KIND=4) DIMENSION(*) for dfsintegrate1d/dfsintegrateex1d

REAL(KIND=8) DIMENSION(*) for dfdintegrate1d/dfdintegrateex1d

C: float* for dfsIntegrate1D/dfsIntegrateEx1D

double* for dfdIntegrate1D/dfdIntegrateEx1D

Array of size nlim that defines the right-side integration limits.

rlimhint

Fortran: INTEGER

C: MKL_INT

A flag describing the structure of the right-side integration limits rlim. For the list of possible values of rlimhint, see table "Hint Values for Integration Limits". If you set the flag to the DF_NO_HINT value, the library assumes that the right-side integration limits define a non-uniform partition.

ldatahint

Fortran: REAL(KIND=4) DIMENSION(*) for dfsintegrate1d/dfsintegrateex1d

REAL(KIND=8) DIMENSION(*) for dfdintegrate1d/dfdintegrateex1d

C: float* for dfsIntegrate1D/dfsIntegrateEx1D

double* for dfdIntegrate1D/dfdIntegrateEx1D

Array that contains additional information about the structure of partition x and left-side integration limits. For details on the ldatahint array, see table "Structure of the datahint Array" in the description of the df?Intepolate1D function.

rdatahint

Fortran: REAL(KIND=4) DIMENSION(*) for dfsintegrate1d/dfsintegrateex1d

REAL(KIND=8) DIMENSION(*) for dfdintegrate1d/dfdintegrateex1d

C: float* for dfsIntegrate1D/dfsIntegrateEx1D

double* for dfdIntegrate1D/dfdIntegrateEx1D

Array that contains additional information about the structure of partition x and right-side integration limits. For details on the rdatahint array, see table "Structure of the datahint Array" in the description of the df?Intepolate1D function.

r

Fortran: REAL(KIND=4) DIMENSION(*) for dfsintegrate1d/dfsintegrateex1d

REAL(KIND=8) DIMENSION(*) for dfdintegrate1d/dfdintegrateex1d

C: float* for dfsIntegrate1D/dfsIntegrateEx1D

double* for dfdIntegrate1D/dfdIntegrateEx1D

Array of integration results. The size of the array should be sufficient to hold nlim*ny values, where ny is the dimension of the vector-valued function. The integration results are packed according to the settings in rhint.

rhint

Fortran: REAL(KIND=4) DIMENSION(*) for dfsintegrate1d/dfsintegrateex1d

REAL(KIND=8) DIMENSION(*) for dfdintegrate1d/dfdintegrateex1d

C: float* for dfsIntegrate1D/dfsIntegrateEx1D

double* for dfdIntegrate1D/dfdIntegrateEx1D

A flag describing the structure of the results. For the list of possible values of rhint, see table "Hint Values for Integration Results". If you set the flag to the DF_NO_HINT value, the library stores the results in row-major format.

le_cb

Fortran: INTEGER

C: dfsIntegrCallBack for dfsIntegrateEx1D

dfdIntegrCallBack for dfdIntegrateEx1D

User-defined callback function for integration on interval [ llim[i], min(rlim[i], a)) for llim[i] < a .

le_params

Fortran: INTEGER DIMENSION(*)

C: void*

Pointer to additional user-defined parameters passed by the library to the le_cb function.

re_cb

Fortran: INTEGER

C: dfsInterpCallBack for dfsIntegrateEx1D

dfdInterpCallBack for dfdIntegrateEx1D

User-defined callback function for integration on interval [max(llim[i], b), rlim[i]) for rlim[i] ≥ b.

re_params

Fortran: INTEGER DIMENSION(*)

C: void*

Pointer to additional user-defined parameters passed by the library to the re_cb function.

i_cb

Fortran: INTEGER

C: dfsIntegrCallBack for dfsIntegrateEx1D

dfdIntegrCallBack for dfdIntegrateEx1D

User-defined callback function for integration on interval [max(a, llim[i], ), min(rlim[i], b)).

i_params

Fortran: INTEGER DIMENSION(*)

C: void*

Pointer to additional user-defined parameters passed by the library to the i_cb function.

search_cb

Fortran: INTEGER

C: dfsSearchCellsCallBack for dfsIntegrateEx1D

dfdSearchCellsCallBack for dfdIntegrateEx1D

User-defined callback function for computing indices of cells that can contain interpolation sites.

search_params

Fortran: INTEGER DIMENSION(*)

C: void*

Pointer to additional user-defined parameters passed by the library to the search_cb function.

Output Parameters

Name

Type

Description

status

Fortran: INTEGER

C: int

Status of the routine:

  • DF_STATUS_OK if the routine execution completed successfully.
  • Non-zero error code if the routine execution failed. See "Task Status and Error Reporting" for error code definitions.

Description

The df?integrate1d/df?integrateex1d routine computes spline-based integral on user-defined intervals

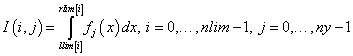
If rlim[i] < llim[i], the routine returns

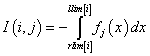
The routine supports the following hint values for integration results:

Hint Values for Integration Results

Value

Description

DF_MATRIX_STORAGE_ROWS

Data is stored in row-major format according to C conventions.

DF_MATRIX_STORAGE_COLS

Data is stored in column-major format according to Fortran conventions.

DF_NO_HINT

No hint is provided. By default, the coordinates of vector-valued function y are provided and stored in row-major format.

A common structure of the storage formats for the integration results is as follows:

Using the llimhint and rlimhint parameters, you can provide the following hint values for integration limits:

Hint Values for Integration Limits

Value

Description

DF_SORTED_DATA

Integration limits are sorted in the ascending order and define a non-uniform partition.

DF_NON_UNIFORM_PARTITION

Partition defined by integration limits is non-uniform.

DF_UNIFORM_PARTITION

Partition defined by integration limits is uniform.

DF_NO_HINT

No hint is provided. By default, partition defined by integration limits is interpreted as non-uniform.

To compute integration with splines unsupported in the Data Fitting component, use the extended version of the routine df?integrateex1d. With this routine, you can provide user-defined callback functions that compute:

If you do not pass a callback function, the routine uses the default settings.

Parent topic: Computational Routines

See Also

df?interpolate1d/df?interpolateex1d
df?integrcallback
df?searchcellscallback

Submit feedback on this help topic